Introduction to Trigonometry
See also: Geometry an IntroductionTrigonometry, as the name might suggest, is all about triangles.
More specifically, trigonometry is about right-angled triangles, where one of the internal angles is 90°. Trigonometry is a system that helps us to work out missing or unknown side lengths or angles in a triangle.
There is more about triangles on our page on Polygons should you need to brush up on the basics before you read further here.
Right-Angled Triangles: A Reminder
A right-angled triangle has a single right angle. By definition, that means that all sides cannot be the same length. A typical right-angled triangle is shown below.
Important Terms for Right-Angled Triangles

-
The right angle is indicated by the little box in the corner.
-
The other angle that we (usually) know is indicated by θ (theta).
The side opposite the right angle, which is the longest side, is called the hypotenuse.
The side opposite θ is called the opposite.
The side next to θ which is not the hypotenuse is called the adjacent.
Pythagoras’ Theorem vs. Trigonometry
Pythagoras was a Greek philosopher who lived over 2500 years ago. He is credited with a number of important mathematical and scientific discoveries, arguably the most significant of which has become known as Pythagoras’ Theorem.
It is an important rule that applies only to right-angled triangles. It says that ‘the square on the hypotenuse is equal to the sum of the squares on the other two sides.’
That sounds rather complicated, but it is actually quite a simple concept when we see it in a diagram:

Pythagoras’ Theorem says :
a2 + b2 = c2
So, if we know the length of two sides of a triangle and we need to calculate the third, we can use Pythagoras’ Theorem.
However, if we know only one side length and one of the internal angles, then Pythagoras is no use to us on its own and we need to use trigonometry.
Introducing Sine, Cosine and Tangent
There are three basic functions in trigonometry, each of which is one side of a right-angled triangle divided by another.
The three functions are:
| Name | Abbreviation | Relationship to sides of the triangle |
| Sine | Sin | Sin (θ) = Opposite/hypotenuse |
| Cosine | Cos | Cos (θ) = Adjacent/hypotenuse |
| Tangent | Tan | Tan (θ) = Opposite/adjacent |
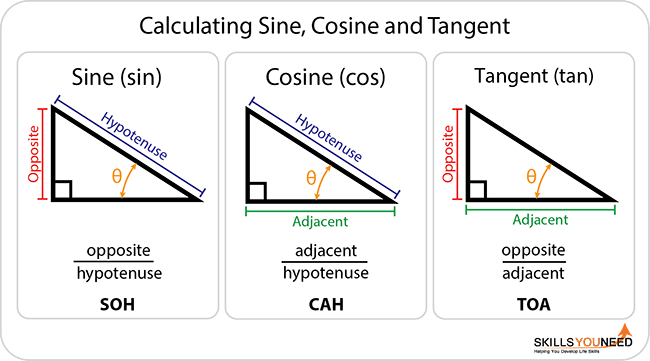
You may find it helpful to remember Sine, Cosine and Tangent as SOH CAH TOA.
Remembering trigonometric functions can be difficult and confusing to begin with. Even SOH CAH TOA can be tricky. You could try making up a funny mnemonic to help you remember. Just keep each group of three letters in the same order.
For example, TOA SOH CAH could be ’The Old Archaeologist Sat On His Coat And Hat’.
Top Tip!
Because of the relationships between them, Tan θ can also be calculated as:
Sin θ / Cos θ.
This means that:
- Sin θ = Cos θ × Tan θ and
- Cos θ = Sin θ / Tan θ.
Trigonometry in a Circle
For more about circles, or a quick refresher, see our page on Circles and Curved Shapes.
When considering triangles, we are limited to angles less than 90°. However, trigonometry is equally applicable to all angles, from 0 to 360°. To understand how the trigonometric functions work with angles greater than 90°, it is helpful to think about triangles constructed within a circle.
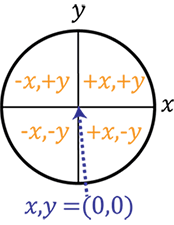
Consider a circle, divided into four quadrants.
Conventionally, the centre of the circle is considered as a Cartesian coordinate of (0,0). That is, the x value is 0 and the y value is 0. For more about this, see our page on Cartesian coordinates.
Anything to the left of the centre has an x value of less than 0, or is negative, while anything to the right has a positive value.
Similarly anything below the centre point has a y value of less than 0, or is negative and any point in the top of the circle has a positive y value.

Diagram i shows what happens if we draw a radius from the centre of the circle to the right along the x axis (we say this is in a positive direction).
We then rotate the radius in an anticlockwise direction through an angle theta θ. This creates a right-angled triangle.
| Sin θ = | opposite (red line) |
| hypotenuse (blue line) |
| Cos θ = | adjacent (green line) |
| hypotenuse (blue line) |
In Diagram ii, we have rotated the radius further in an anti-clockwise direction, past the vertical (y axis) into the next quadrant. Here θ is an obtuse angle, between 90° and 180°. The reference angle alpha α is equal to 180° − θ, and is the acute angle within the right-angled triangle.
| Sin θ = Sin α = | opposite (red line) |
| hypotenuse (blue line) |
Both the blue and red lines are positive, so sin θ is positive.
| Cos θ = −Cos α = | adjacent (green line) |
| hypotenuse (blue line) |
Cos θ is negative, since the green line is negative (it lies along the x axis to the left of the origin (0,0), so is in the negative section of the x axis).
In Diagram iii, the radius has rotated further anticlockwise into the next quadrant so that the value of θ is between 180° and 270°. The green, red and blue lines all have negative values and α = θ − 180°. Sines and cosines are therefore all positive in value.
Diagram iv shows the final quadrant. The value of θ is between 270° and 360°, the green line is positive, but the red and blue lines are negative. Sin θ is therefore positive and Cos θ is negative. α = 360° − θ.
The Unit Circle
The 'Unit Circle' is a special case of the circle shown in the diagrams above. The Unit Circle has a radius of 1.
When working with a unit circle we can measure cos, sin and tan directly:

Graphs of Sine, Cosine and Tangent
The relationship between the angle and the sin or cos can be drawn as a graph:
- y = sin (θ)
- y = cos (θ)

You can see that when θ is 0, then so is sine. This makes sense when you look at the unit circle diagram above. When θ = 0, the adjacent and hypotenuse both lie along the positive x axis and the red line that shows the value of sin θ disappears (there is no triangle).
The cosine graph is the same shape to sine, but has a value of 1 when θ = 0. Looking again at the circle above, when θ = 0, the adjacent and hypotenuse both lie along the positive x axis and have the same value, so adjacent/hypotenuse = 1.
The cyclic nature of the sine and cosine graphs is incredibly important throughout science, nature and engineering. Examples include electrical applications (alternating current), sound and radio waves, simple harmonic motion (such as a swinging pendulum), the trajectory of satellites, or the rising and falling of the tide.
The amplitude of a cyclic wave pattern is the value of the ‘peak’ in the graph, i.e. the distance from the x-axis to the maximum or minimum value. In the Sine and Cosine graphs above, the amplitude has a value of 1. In applications such as sound or electrical current, the amplitude varies, depending on the volume of the sound or magnitude of the current. The amplitude of the tides also varies, depending on the position of the moon and its ‘pull’ on the earth.
The characteristics of the tangent graph (tan θ) are quite different. The tangent graph does not have an amplitude (wave-like characteristics) because it has no maximum or minimum peak values. It changes from −∞ to +∞ (negative and positive infinity) crossing through 0 every 180°:
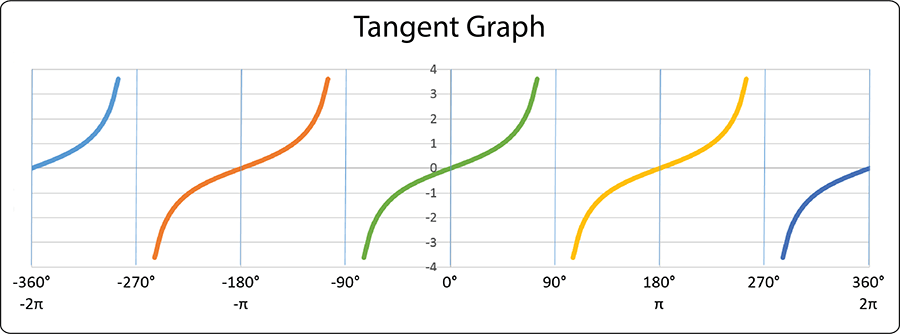
At infinity (positive or negative) it is said to be undefined. We can understand this graph better when we consider the equation tan θ = sin θ / cos θ. Whenever sin θ is zero, then tan θ must also be zero. Conversely, whenever cos θ is zero, then the denominator in the equation becomes zero. Anything divided by zero has a value of infinity, so the values of θ that have a cosine of zero also have a tangent of infinity on the graph. Infinity doesn’t have an exact value, so the lines on the tangent graph become more and more vertical as the y axis increases to greater and greater values. The lines get closer and closer to the vertical lines on the graph for particular values of θ, for example at 90°. Each of these vertical lines is called an asymptote.
Inverse of Sine, Cosine and Tangent
You can also work out the inverse function to sin, cos and tan, which means 1 divided by that function. They are designated as sin/cos/tan -1. This enables you to work out the angle if you have the sin, cos or tan of it.
In other words:
- Sin (90) = 1
- Sin−1 (1) = 90°
Trigonometry and Calculators
Scientific calculators have sin, cos, and tan functions, as well as inverse functions. It’s worth taking a few minutes to work out how your calculator operates, as this could save you hours of messing about when you need it.
Other Triangles and Trigonometry
Trigonometry also works for other triangles, just not in quite the same way. Instead there are two rules based on a triangle like this:
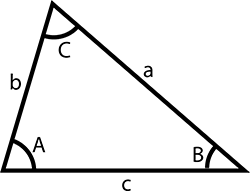
The Sine rule is:
a/sin A = b/sin B = c/sin C
The Cosine Rule is:
c2 = a2 + b2 – 2ab cos (C)
Why Do I Need Trigonometry?
This is a reasonable question, and the answer is at least partly because those who decide the mathematics curriculum in many countries think that you should know about it, and for very good reason.
Trigonometry is said to be the most important mathematical relationship ever discovered. Triangles are one of the most simple forms found in nature, but their mathematics has vital importance, especially where precise distance measurements are needed. When we begin to think about the applications where accurate distances are important, it is apparent that there are dozens, including navigation in naval and aviation systems, astronomy, satellite systems, geographical surveys and cartography (maps), architecture and structural engineering, graphic design and computer generated imagery.
Many of these rely on a measurement technique known as triangulation, which applies the concepts of trigonometry.
Example: Trigonometry and Navigation
When you are sailing or cruising at sea, where you end up is affected by:
- The direction in which you steer;
- The speed at which you travel in that direction (i.e. the motor or wind speed); and
- The direction and speed of the tide.
You can be motoring in one direction, but the tide could be coming from one side, and push you to the other. You will need trigonometry to work out how far you will travel and in what precise direction.

You will, quite rightly, have worked out that it’s not quite as simple as all that, because the actual direction of travel depends on the tide speed and your speed, but you can probably see why trigonometry might be important!
Worked Example
You are out for a day’s sailing, and don’t really mind where you end up. You started out heading due east, and plan to sail for one hour at a cruising speed of 10 km/h. The tide is due north, and running at 5km/h. What direction will you end up travelling in?
First draw your triangle, and label the sides. You are heading due east, so let’s make that the bottom of the triangle, length 10km. The tide is going to push you north, so let’s make that the right hand side. And you want to know what direction you’ll end up going in, so that’s angle θ.

You have the opposite and the adjacent, which means that you need to use tangent. Tan θ = Opposite/adjacent = 5/10 = 0.5.
Now is the time to use the inverse tan function. The inverse tan of 0.5 is 26.6°. In other words, tan 26.6 = 0.5.
- Direction (your ‘heading’ in navigation) is measured from North, which is 0° on your compass. Your answer from (3), however, is measured from 90°, or East. You will therefore need to subtract your answer from 90°, to obtain the answer: You are travelling in a direction (heading) of 63.4°, which is between North East (45°) and East North East (67.5°).

Why is this important? You’ll need to know which direction you travelled in order to sail home, of course!
In real life, you will also need to remember that by then the tide may have changed…
Conclusion
Trigonometry may not have all that many everyday applications, but it does help you to work with triangles more readily. It’s a useful supplement to geometry and actual measurements, and as such well worth developing an understanding of the basics, even if you never wish to progress further.
Continue to:
Geometry
Introduction to Algebra
More numeracy skills:
Systems of Measurement | Maths Glossary - Common Symbols
Positive and Negative Numbers | Graphs and Charts
